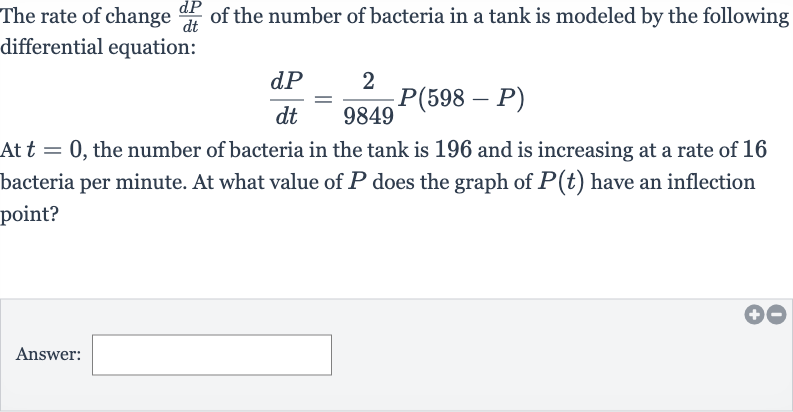
The rate of change $\frac{d P}{d t}$ of the number of bacteria in a tank is modeled by the following differential equation: $\newline$ $\frac{d P}{d t}=\frac{2}{9849} P(598-P)$ $\newline$ At $t=0$ , the number of bacteria in the tank is $196$ and is increasing at a rate of $16$ bacteria per minute. At what value of $P$ does the graph of $P(t)$ have an inflection point? $\newline$ Answer:

View step-by-step help

Home

Math Problems

Precalculus

Find instantaneous rates of change

Full solution

Q. The rate of change

\frac{d P}{d t}

of the number of bacteria in a tank is modeled by the following differential equation:

\newline

\frac{d P}{d t}=\frac{2}{9849} P(598-P)

\newline

t=0

, the number of bacteria in the tank is

196

and is increasing at a rate of

16

bacteria per minute. At what value of

P

does the graph of

P(t)

have an inflection point?

\newline

Answer:

Find First Derivative: To find the inflection point, we need to find the second derivative of $P$ with respect to $t$ and set it equal to zero. The second derivative will tell us where the concavity of the graph changes, which is the inflection point. $\newline$ First, let's find the first derivative, which is given by the differential equation: $\newline$ $\frac{dP}{dt} = \frac{2}{9849}P(598 - P)$
Find Second Derivative: Now, we need to find the second derivative $\frac{d^2P}{dt^2}$ . To do this, we will differentiate $\frac{dP}{dt}$ with respect to $P$ and then multiply by $\frac{dP}{dt}$ again due to the chain rule. $\newline$ $\frac{d^2P}{dt^2} = \frac{d}{dP}\left(\frac{dP}{dt}\right) \times \frac{dP}{dt}$
Differentiate First Derivative: Differentiating $\frac{dP}{dt}$ with respect to $P$ gives us: $\frac{d}{dP}\left(\frac{2}{9849}P(598 - P)\right) = \frac{2}{9849}(598 - 2P)$
Multiply Derivatives: Now, we multiply this derivative by $(\frac{dP}{dt})$ to get the second derivative with respect to time: $(\frac{d^2P}{dt^2}) = (\frac{2}{9849})(598 - 2P) \times (\frac{2}{9849})P(598 - P)$
Set Second Derivative Equal to Zero: To find the inflection point, we set the second derivative equal to zero and solve for $P$ : $0 = \left(\frac{2}{9849}\right)\left(598 - 2P\right) * \left(\frac{2}{9849}\right)P\left(598 - P\right)$
Simplify Equation: We can simplify the equation by setting the factors equal to zero: $\newline$ $598 - 2P = 0$ or $P(598 - P) = 0$
Solve for P: Solving the first equation for P gives us: $\newline$ $2P = 598$ $\newline$ $P = 299$
Disregard Endpoints: Solving the second equation for $P$ gives us two solutions: $\newline$ $P = 0$ or $598 - P = 0$ $\newline$ $P = 0$ or $P = 598$
Identify Inflection Point: However, since we are looking for the inflection point where $P$ is changing, we disregard the solutions $P = 0$ and $P = 598$ because they represent the endpoints of the interval where the population exists. The inflection point occurs at $P = 299$ .