Full solution
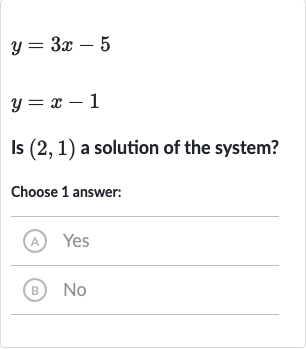
Q. Is a solution of the system?Choose answer:(A) Yes(B) No
- Substitute and Check First Equation: First, we will substitute the point into the first equation and check if it holds true. The first equation is . If we substitute and , we get .
- Substitute and Check Second Equation: After performing the calculation, we find that , which simplifies to , which is true. Therefore, the point satisfies the first equation.
- Point Satisfies Both Equations: Next, we will substitute the point into the second equation and check if it holds true. The second equation is y = x - 1 \. If we substitute \$ x=2 and , we get \(1 = - \.
- Point Satisfies Both Equations: Next, we will substitute the point into the second equation and check if it holds true. The second equation is . If we substitute and , we get . After performing the calculation, we find that , which is also true. Therefore, the point satisfies the second equation as well.
- Point Satisfies Both Equations: Next, we will substitute the point into the second equation and check if it holds true. The second equation is . If we substitute and , we get . After performing the calculation, we find that , which is also true. Therefore, the point satisfies the second equation as well. Since the point satisfies both equations, it is a solution to the system of equations.
More problems from Is (x, y) a solution to the system of equations?
QuestionGet tutor help
QuestionGet tutor help