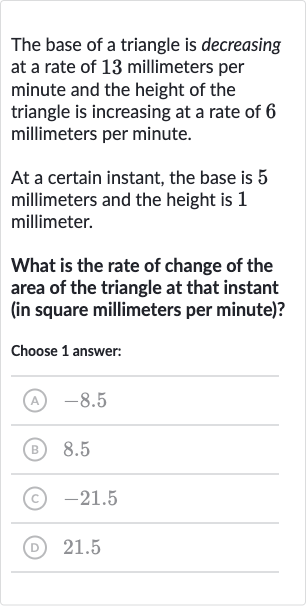
The base of a triangle is decreasing at a rate of $13$ millimeters per minute and the height of the triangle is increasing at a rate of $6$ millimeters per minute. $\newline$ At a certain instant, the base is $5$ millimeters and the height is $1$ millimeter. $\newline$ What is the rate of change of the area of the triangle at that instant (in square millimeters per minute)? $\newline$ Choose $1$ answer: $\newline$ (A) $-8$ . $5$ $\newline$ (B) $8$ . $5$ $\newline$ (C) $-21$ . $5$ $\newline$ (D) $21$ . $5$

Full solution

Q. The base of a triangle is decreasing at a rate of

13

millimeters per minute and the height of the triangle is increasing at a rate of

6

millimeters per minute.

\newline

At a certain instant, the base is

5

millimeters and the height is

1

millimeter.

\newline

What is the rate of change of the area of the triangle at that instant (in square millimeters per minute)?

\newline

Choose

1

answer:

\newline

(A)

-8

5

\newline

(B)

8

5

\newline

(C)

-21

5

\newline

(D)

21

5

Area Formula: The area of a triangle is given by the formula $A = \frac{1}{2} \times \text{base} \times \text{height}$ .
Derivative Calculation: Let's denote the base by $b$ and the height by $h$ . The rate of change of the area with respect to time $t$ can be found using the derivative $\frac{dA}{dt} = \frac{1}{2} \cdot (\frac{db}{dt} \cdot h + b \cdot \frac{dh}{dt})$ .
Given Rates: Given $\frac{db}{dt} = -13$ mm/min (since the base is decreasing) and $\frac{dh}{dt} = 6$ mm/min (since the height is increasing).
Instant Values: At the instant when the base $b$ is $5\,\text{mm}$ and the height $h$ is $1\,\text{mm}$ , we plug these values into the derivative formula to find $\frac{dA}{dt}$ .
Calculate $\frac{dA}{dt}$ : So, $\frac{dA}{dt} = \frac{1}{2} \times (-13 \times 1 + 5 \times 6)$ .
Final Result: Calculating the above expression gives $\frac{dA}{dt} = \frac{1}{2} \times (-13 + 30)$ .
Final Result: Calculating the above expression gives $\frac{dA}{dt} = \frac{1}{2} \times (-13 + 30)$ . $\frac{dA}{dt} = \frac{1}{2} \times 17 = 8.5$ square millimeters per minute.