AI tutor
Full solution
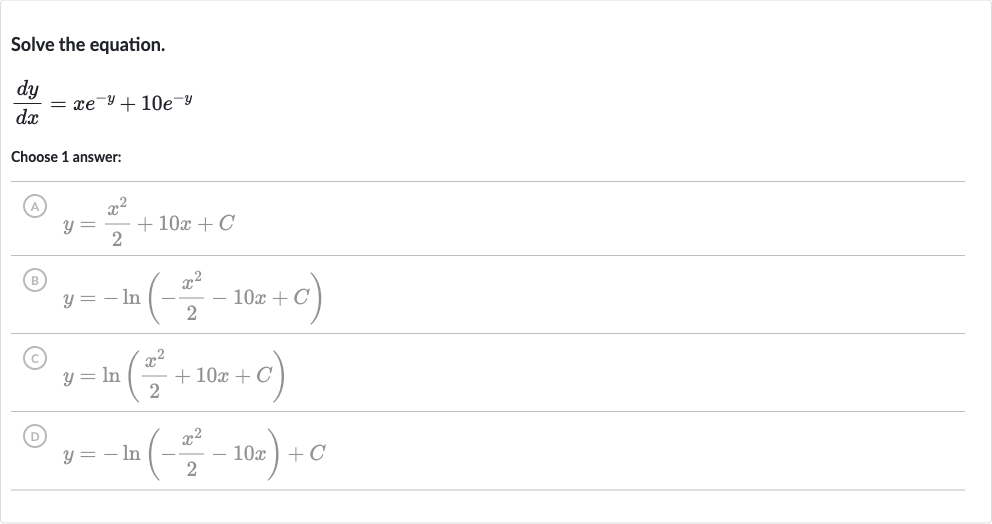
Q. Solve the equation.Choose answer:(A) (B) (C) (D)
- Recognize Separable Equation: Recognize that the given differential equation is separable, meaning we can separate the variables and on different sides of the equation.We can write this as .
- Integrate with Respect: Integrate both sides of the equation with respect to their respective variables.
- Perform Integration: Perform the integration.The integral of with respect to is , and the integral of with respect to is .So we have , where is the constant of integration.
- Solve for y: Solve for y by taking the natural logarithm of both sides.
- Check Answer Choices: Check the answer choices to see which one matches our solution.The correct answer is (C) .