Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

g(x)=15-4x h(x)=(3)/(2)x+8 Write g(h(x)) as an expression in terms of x. g(h(x))=

g(x)=15−4x g(x)=15-4 x \newlineh(x)=32x+8 h(x)=\frac{3}{2} x+8 \newlineWrite g(h(x)) g(h(x)) as an expression in terms of x x .\newlineg(h(x))= g(h(x))=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Is (x, y) a solution to the system of equations?right chevron icon

Full solution

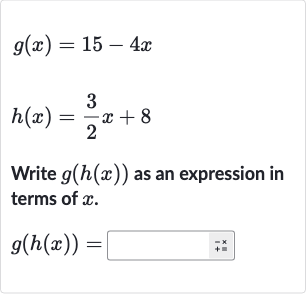
Q. g(x)=15−4x g(x)=15-4 x \newlineh(x)=32x+8 h(x)=\frac{3}{2} x+8 \newlineWrite g(h(x)) g(h(x)) as an expression in terms of x x .\newlineg(h(x))= g(h(x))=
  1. Substitute h(x)h(x) into g(x)g(x): To find g(h(x))g(h(x)), we need to substitute the expression for h(x)h(x) into the function g(x)g(x).
    g(x)=15−4xg(x) = 15 - 4x
    h(x)=(32)x+8h(x) = (\frac{3}{2})x + 8
    Substitute h(x)h(x) into g(x)g(x):
    g(h(x)) = \(15 - 44[(\frac{33}{22})x + 88]
  2. Distribute −4-4 inside the brackets: Now, distribute the −4-4 inside the brackets to both terms in the expression (32)x+8(\frac{3}{2})x + 8.\newlineg(h(x))=15−4[(32)x]−4[8]g(h(x)) = 15 - 4[(\frac{3}{2})x] - 4[8]\newline=15−6x−32= 15 - 6x - 32
  3. Combine constant terms: Combine the constant terms 1515 and −32-32.g(h(x))=15−32−6x=−17−6xg(h(x)) = 15 - 32 - 6x = -17 - 6x

More problems from Is (x, y) a solution to the system of equations?

Question
Solve using substitution. 5x−2y=−7 5x - 2y = -7 x=−5 x = -5 (_,_)
Get tutor helpright-arrow

Posted 10 months ago

Question
Is (1,1)(1,1) a solution to this system of equations? \newline4x+10y=144x + 10y = 14\newlinex+6y=7x + 6y = 7\newlineChoices:\newline(A) yes \newline(B) no
Get tutor helpright-arrow

Posted 10 months ago

Question
Which describes the system of equations below?\newliney=–3x+9y = –3x + 9 \newliney=–3x+9y = –3x + 9 \newlineChoices:\newline(A) consistent and independent\text{(A) consistent and independent} \newline(B) consistent and dependent\text{(B) consistent and dependent} \newline(C) inconsistent\text{(C) inconsistent}
Get tutor helpright-arrow

Posted 10 months ago

Question
Solve using elimination. \newline7x−8y=−17 7x - 8y = -17 \newline −7x+3y=2 -7x + 3y = 2 \newline (____,____)(\_\_\_\_, \_\_\_\_)
Get tutor helpright-arrow

Posted 10 months ago

Question
Solve. \newlinex=−2 x = -2 \newline−2x+2y=−8 -2x + 2y = -8 \newline(____,____)(\_\_\_\_, \_\_\_\_)
Get tutor helpright-arrow

Posted 1 year ago

Question
Write a system of equations to describe the situation below, solve using any method, and fill in the blanks.\newlineAt a community barbecue, Mrs. Wilkerson and Mr. Hogan are buying dinner for their families. Mrs. Wilkerson purchases 33 hot dog meals and 33 hamburger meals, paying a total of $36\$36. Mr. Hogan buys 11 hot dog meal and 33 hamburger meals, spending $26\$26 in all. How much do the meals cost?\newlineHot dog meals cost $\$_______ each, and hamburger meals cost $\$________ each.
Get tutor helpright-arrow

Posted 1 year ago

Question
Solve the system of equations by substitution.\newline−3x−y−3z=−11-3x - y - 3z = -11\newlinez=5z = 5\newlinex−y+3z=19x - y + 3z = 19\newline(____.____,____)
Get tutor helpright-arrow

Posted 10 months ago

Question
Solve the system of equations by elimination.\newlinex−3y−2z=10 x - 3y - 2z = 10 \newline3x+2y+2z=14 3x + 2y + 2z = 14 \newline2x−3y−2z=16 2x - 3y - 2z = 16 \newline(_,_,_)(\_,\_,\_)
Get tutor helpright-arrow

Posted 10 months ago

Question
Solve the system of equations.\newliney=x2+36x+3 y = x^2 + 36x + 3 \newliney=22x−37 y = 22x - 37 \newlineWrite the coordinates in exact form. Simplify all fractions and radicals.\newline(_,_)(\_,\_)\newline(_,_)(\_,\_)
Get tutor helpright-arrow

Posted 10 months ago

Question
Solve the system of equations.\newliney=−x−24 y = -x - 24 \newlinex2+y2=488 x^2 + y^2 = 488 \newlineWrite the coordinates in exact form. Simplify all fractions and radicals.\newline(_,_) (\_,\_) \newline(_,_) (\_,\_)
Get tutor helpright-arrow

Posted 10 months ago

View all (74)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant