Express as a function of a DIFFERENT angle, 0^(@)

Question

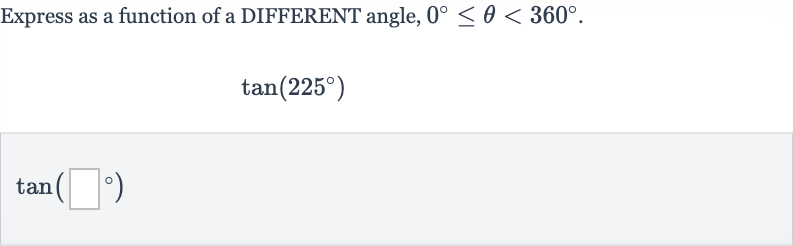
Express as a function of a DIFFERENT angle,  0^(@) <= theta < 360^(@).  tan(225^(@))  tan(◻^(@))

Bytelearn · Accepted Answer

Understand Problem and Range: Understand the problem and the range for θ. We need to express tan(225°) in terms of another angle that is within the range of 0° to 360°. Since 225° is outside this range, we need to find an equivalent angle within the range.Find Reference Angle: Find the reference angle for 225°. The reference angle is the acute angle that the terminal side of the given angle makes with the x-axis. Since 225° is in the third quadrant, its reference angle is 225° - 180° = 45°.Determine Tangent Sign: Determine the sign of the tangent function in the third quadrant. In the third quadrant, both sine and cosine are negative, so the tangent (which is sine divided by cosine) is positive. Therefore, tan(225°) will have the same value as tan(45°) but with a positive sign.Express as Function of Reference Angle: Express tan(225°) as a function of the reference angle. Since the reference angle is 45° and tan(45°) = 1, we can express tan(225°) as tan(45°). However, we need to find a different angle, not the reference angle itself.Find Angle with Same Tangent Value: Find a different angle with the same tangent value. We know that the tangent function has a period of 180°, so if we add or subtract multiples of 180° to 45°, we will get angles with the same tangent value. The closest angle within the range of 0° to 360° that is not 45° is 45° + 180° = 225°, but this is the original angle given. The next angle would be 45° - 180°, but this is negative and outside the range. Therefore, we need to look for another approach.Use Tangent Symmetry: Use the symmetry of the tangent function. The tangent function is also symmetrical about the origin, which means that tan(θ) = -tan(180° + θ). So, we can find an angle θ such that 180° + θ = 225°. Solving for θ gives us θ = 225° - 180° = 45°, but again, this is the reference angle itself.Correct Approach: Realize the mistake and correct the approach. We made a mistake in the previous steps by not finding a different angle. We need to find an angle that is coterminal with 225° and within the range of 0° to 360°. Since 225° is already within the range, we need to find an angle that has the same tangent value but is not the same as 225° or its reference angle of 45°.

Express as a function of a DIFFERENT angle, 0^{\circ} \leq \theta<360^{\circ} . $\newline$ $\tan \left(225^{\circ}\right)$ $\newline$ $\tan \left(\square^{\circ}\right)$

Full solution

More problems from Find trigonometric ratios using reference angles

Related topics

Express as a function of a DIFFERENT angle, 0^{\circ} \leq \theta<360^{\circ} .\newlinetan⁡(225∘) \tan \left(225^{\circ}\right) tan(225∘)\newlinetan⁡(□∘) \tan \left(\square^{\circ}\right) tan(□∘)

Express as a function of a DIFFERENT angle, 0^{\circ} \leq \theta<360^{\circ} . $\newline$ $\tan \left(225^{\circ}\right)$ $\newline$ $\tan \left(\square^{\circ}\right)$