Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

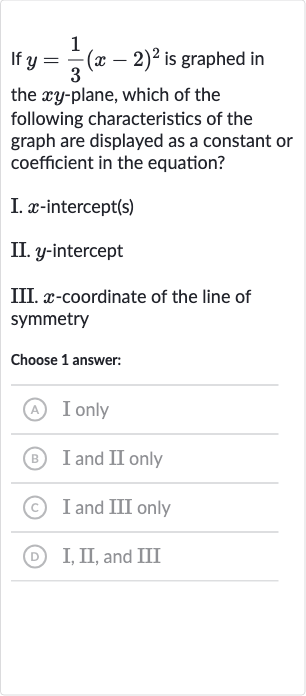
If y=(1)/(3)(x-2)^(2) is graphed in the xy-plane, which of the following characteristics of the graph are displayed as a constant or coefficient in the equation? I. x-intercept(s) II. y-intercept III. x-coordinate of the line of symmetry Choose 1 answer: (A) I only (B) I and II only (C) I and III only (D) I, II, and III

If y=13(x−2)2 y=\frac{1}{3}(x-2)^{2} is graphed in the xy x y -plane, which of the following characteristics of the graph are displayed as a constant or coefficient in the equation?\newlineI. x x -intercept(s)\newlineII. y y -intercept\newlineIII. x x -coordinate of the line of symmetry\newlineChoose 11 answer:\newline(A) I only\newline(B) I and II only\newline(C) I and III only\newline(D) I, II, and III

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Domain and rangeright chevron icon

Full solution

Q. If y=13(x−2)2 y=\frac{1}{3}(x-2)^{2} is graphed in the xy x y -plane, which of the following characteristics of the graph are displayed as a constant or coefficient in the equation?\newlineI. x x -intercept(s)\newlineII. y y -intercept\newlineIII. x x -coordinate of the line of symmetry\newlineChoose 11 answer:\newline(A) I only\newline(B) I and II only\newline(C) I and III only\newline(D) I, II, and III
  1. Analyze Equation Characteristics: Analyze the given equation y=13(x−2)2y=\frac{1}{3}(x-2)^2 to determine the characteristics of its graph.
  2. Line of Symmetry: The xx-coordinate of the line of symmetry for a parabola in the form y=a(x−h)2+ky=a(x-h)^2+k is hh. In the given equation, h=2h=2, which means the line of symmetry is x=2x=2. This is directly given by the coefficient in the equation.
  3. Y-Intercept Calculation: The y-intercept occurs when x=0x=0. To find the y-intercept, substitute x=0x=0 into the equation and solve for yy.y=(13)(0−2)2y=\left(\frac{1}{3}\right)(0-2)^2y=(13)(−2)2y=\left(\frac{1}{3}\right)(-2)^2y=(13)(4)y=\left(\frac{1}{3}\right)(4)y=43y=\frac{4}{3}The y-intercept is not directly given as a constant or coefficient in the equation; it is a result of evaluating the function at x=0x=0.
  4. X-Intercept Calculation: The xx-intercepts occur when y=0y=0. Set the equation equal to zero and solve for xx. \newline0=(13)(x−2)20=\left(\frac{1}{3}\right)(x-2)^2\newlineSince (x−2)2(x-2)^2 is always non-negative, the only solution for this equation is when x−2=0x-2=0, which gives x=2x=2. This means there is only one xx-intercept, and it coincides with the line of symmetry. However, the xx-intercept is not displayed as a constant or coefficient in the equation; it is a result of solving the equation when y=0y=0.

More problems from Domain and range

Question
What is the domain of this function?\newline(9,–2)(6,–10)(–3,9)(5,2)(9,–2)(6,–10)(–3,9)(5,2)\newlineChoices:\newline(A){–3,5,6,9}(B){–10,–2,2,9}(C){2,5,6,9}(D){–6,–3,5,9}(A)\{–3,5,6,9\}\newline(B)\{–10,–2,2,9\}\newline(C)\{2,5,6,9\}\newline(D)\{–6,–3,5,9\}
Get tutor helpright-arrow

Posted 1 year ago

Question
Look at this set of ordered pairs:\newline(2,14)(2, 14)\newline(10,12)(10, 12)\newline(3,−19)(3, -19)\newlineIs this relation a function?\newlineChoices:\newline[[yes]\text{[[yes]}[no]]\text{[no]]}
Get tutor helpright-arrow

Posted 1 year ago

Question
Use the following function rule to find f(11) f(11) .\newlinef(x)=−10−7x f(x) = -10 - 7x \newlinef(11)= f(11) = _____
Get tutor helpright-arrow

Posted 1 year ago

Question
Find the slope of the line y=−3x−25 y = -3x - \frac{2}{5} .\newlineSimplify your answer and write it as a proper fraction, improper fraction, or integer.\newline_____\_\_\_\_\_
Get tutor helpright-arrow

Posted 1 year ago

Question
A line has a slope of 33 and passes through the point (−2,−10)(-2,-10). Write its equation in slope-intercept form.\newlineWrite your answer using integers, proper fractions, and improper fractions in simplest form.
Get tutor helpright-arrow

Posted 1 year ago

Question
What is the range of this function?\newliney = |x|\newlineChoices:\newlineall real numbers\text{all real numbers}\newline{y∣y≥0}\{y \mid y \geq 0\}\newline{y∣y≤0}\{y \mid y \leq 0\}\newline{y∣y>0}\{y \mid y > 0\}
Get tutor helpright-arrow

Posted 1 year ago

Question
Find g(x) g(x) , where g(x) g(x) is the translation 1 1 unit down of f(x)=∣x∣ f(x) = |x| .\newlineWrite your answer in the form a∣x−h∣+k a|x - h| + k , where a a , h h , and k k are integers.\newlineg(x)= g(x) = ______\newline
Get tutor helpright-arrow

Posted 1 year ago

Question
What is the domain of this function?\newline(8,–1)(7,–11)(–4,10)(4,3)(8,–1)(7,–11)(–4,10)(4,3)\newlineChoices: \newline(A){–4,4,7,8}(A)\{–4,4,7,8\}\newline(B){–11,–1,3,10}(B)\{–11,–1,3,10\}\newline(C){3,4,7,8}(C)\{3,4,7,8\}\newline(D){–7,–4,4,8}(D)\{–7,–4,4,8\}
Get tutor helpright-arrow

Posted 1 year ago

Question
What is the domain of this function?\newline(10, –3)(2, –9)(–5, 8)(3, 1)(10,\,–3)(2,\,–9)(–5,\,8)(3,\,1)\newlineChoices:\newline[{–5,2,3,10}][{–9, –3,1,8}][{1,2,3,10}][{–10, –5,2,3}][\{–5,2,3,10\}][\{–9,\,–3,1,8\}][\{1,2,3,10\}][\{–10,\,–5,2,3\}]
Get tutor helpright-arrow

Posted 1 year ago

Question
What is the domain of this function?\newline(1,–4)(9,–12)(–6,11)(7,5)(1,–4)(9,–12)(–6,11)(7,5)\newlineChoices:(A){–6,1,7,9}\newline\\(A)\{–6,1,7,9\}\newline(B){–12,–4,5,11}(B)\{–12,–4,5,11\}\newline(C{5,1,7,9}(C\{5,1,7,9\}\newline(D){–9,–6,1,7}(D)\{–9,–6,1,7\}
Get tutor helpright-arrow

Posted 1 year ago

View all (377)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant